In this section it is proposed to describe boiling under steady-state conditions in a vertical, uniformly heated tube. Each of the various heat transfer regions present will be described briefly, together with simple correlations for the estimation of heat transfer coefficients in each region and for the transition from one region to another.

A. Regions of heat transfer in a vertical heated tube

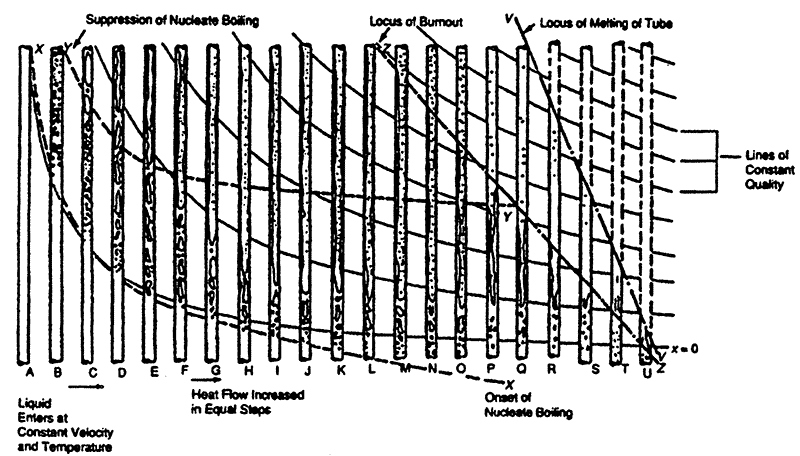
The various regions of flow and heat transfer which occur in the forced convective evaporation of a liquid in a vertical tube are illustrated in Figure 1 (also given in Section 154). This Figure shows how the heat transfer regime and the two phase flow pattern vary along the tube as the heat input is increased in equal steps. The heat input in step A is just sufficient to bring the liquid entering the tube to its saturation temperature. Step B has twice the heat input of Step A, Step C has three times the heat input of Step A etc. The main features seen in Figure 1 are as follows:

1. Lines of constant thermodynamic quality x are shown. Quality is normally defined as the ratio of the vapour mass flux ṁ_g to the total mass flux ṁ. However, in the case of a channel in which evaporation is occurring, quality is defined on the basis of a heat balance and on the assumption of thermodynamic equilibrium. The local enthalpy h at distance z alone the channel can be calculated from the expression:

   \[\label{eq1} h=h_i+\dfrac{P}{\dot{m}S}\int\limits_{o}^{z}\dot{q}dz=xh_g+(1-x)h_\ell \tag{1}\]

   where h_i is the enthalpy of the inlet fluid, P the tube periphery (= π D for a circular tube), S is the tube cross sectional area (= π D ²/4 for a circular tube), q̇ is the heat flux at distance z and h_g and h_ℓ are the saturated enthalpies for the vapour and liquid respectively. Local quality is given by

   \[\label{eq2} x=\dfrac{h-h_\ell}{h_g-h_\ell}=\dfrac{h-h_\ell}{\varDelta h_v} \tag{2}\]

   Note that x can be negative when h < h_ℓ (subcooled conditions) and x > 1 when h > h_g (superheated conditions).

2. Line XX in Figure 1 represents the onset of nucleate boiling at the channel wall. This occurs above the line x = 0 for low heat fluxes and below this line (subcooled conditions) at high heat fluxes.
   
   
3. The generation of vapour within the liquid gives rise to a two-phase flow. The form of this vapour-liquid flow changes from bubble flow through slug flow and chum flow to annular flow and, after the liquid film on the wall dries out, to dispersed droplet flow. A more detailed discussion of two-phase flow regimes is given in Section 154; the important point about the evaporation case is that the regimes occur successively along the channel and exist over relatively short distances. This means that the two-phase flows occurring in evaporation are rarely fully developed.
   
   
4. At high qualities, the heat transfer rate by direct forced convection through the liquid film may become so great that it is impossible to maintain a wall temperature high enough to sustain nucleate boiling. The evaporation process is then one of heat transfer from the wall to the film interface where vapour is generated. Line YY on Figure 1 represents the locus of such suppression of nucleate boiling and nucleate boiling only exists, therefore, in the region between lines XX and YY.
   
   
5. The processes of droplet entrainment and evaporation from the liquid film eventually result in the film drying out (line 22 on Figure 1). The occurrence of dryout is delayed by the redeposition of droplets onto the film. When, after dryout, the wall is no longer wetted by the liquid, the wall temperature increases in the post-dryout region. The transition between the wetted and non-wetted regions is an important one and has been given several names in the literature [critical heat flux (CHF), burnout, dryout, boiling crisis, boiling transition, etc.]. Here, for reasons explained later, we adopt the term critical heat flux (CHF) to denote the phenomenon.
   
   
6. As the heat flux increases beyond the onset of CHF (dryout), the wall temperature in the post-dryout region increases and, eventually, the end region of the tube would melt (line VV on Figure 1). Thus the post-dryout region can exist only in the zone between lines ZZ and VV and the distance between these lines diminishes as heat flux increases. Note also that the CHF transition occurs in the subcooled region at the highest heat fluxes; here, the mechanism is akin to that in pool boiling where a vapour layer is formed separating the wall from the (mainly liquid) cone.

Figure 1 Regions of flow and heat transfer in the evaporation of a liquid in a tube (Hewitt et al., 1994)